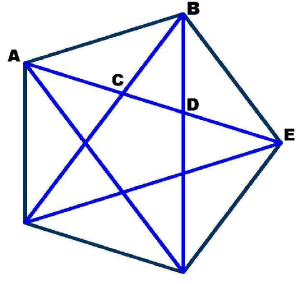
The pentagrams blossomed in 1998 - although one had first appeared in '93 www.lucypringle.co.uk/photos/1993/uk1993de.shtml - and croppies had to start studying golden-ratio geometry. All proportions of the pentagram express this, the 'divine proportion or 'golden ratio,' phi,  . If a pentagon surrounds a pentagram, as shown,  is in the ratio of the sides of each of them, i.e. by AE/AB, AB/BD and BD/CD.  has the value of 1.618… 

A half-turn rotation of a pentagram appeared in 1998, together with a scale-factor enlargement given by half of the golden ratio, /2. This had the effect of setting up a surrounding implied or latent pentagon, just touching the ten corners. That has been a quite distinctive feature of Circlemaker art. 

The enlargement scale- factor is 24%. To ascertain this scale-factor, one has to draw in a right-angled triangle which forms the one-tenth angle at the centre of the pentagram, and reaches out to touch two adjacent corners; then the cosine gives the ratio in size of the two pentagrams, roughly 4:5. It happens that cos /5 = /2 (NB Equations having both pi and phi are rather special, as they are both 'transcendent' numbers which go on forever and never repeat.) where /5 is one-tenth of a circle or 36°. 

A pentagon coincided with the main pentagram, which meant that the angles at the corners had been trisected, i.e. each was 108°, comprising three lots of 36° angles. 

The central circle seemed to indicate a latent pentagram, just fitting into the larger one. This pentagram has to be shrunk by the square of the golden ratio to just touch the inside of the big one.

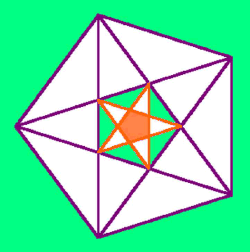A similar scale-factor here applies, where an invisible pentagram is the framework, and six little ones are reduced as the square of the golden ratio. From the outlying pentagon, an inner one is reduced by ², and finally the inmost pentagon is reduced by ⁴ as compared to the outer one. NB, there is a humorous touch in this formation, of an error in flattening too much wheat at one triangle, with a different kind of error in an adjacent one, as if a triangle had been wrongly dropped. These triangles are sometimes called 'divine triangles' with 36° at the apex and side-lengths in the golden ratio. 

I will always remember walking into this lovely formation, with the summer barley blowing and rustling in the wind: one could see the five crescents a-swirling at the centre, but not the overall design. That only appeared from up in the sky! There were five interlocking crescents. Drawing a line bisecting one of these crescents, one found that it touched the tips of all the others. That seemed clever. Then, drawing in all five of these made a big pentagram, the framework for this formation. The inner pentagram marked the centres of the five circles, shown in the diagram by Allan Brown. See if you can construct a second pentagram inside the first, to define the central circle marked by the five small crescents. Can you show that its magnitude is smaller than around the outlying pentagon, by the fourth power of the golden ratio? 

'It was like a kaleidoscope of shimmering fragments and I realised that the 36° angle, the generator of the Golden Section proportion, was repeated no less than 45 times,' to quote Michael Glickmann.

This formation has a pentagram inscribed within a pentagram, which means, as we have seen, that their proportions are as the square of the Golden Ratio. Five smaller ones are cleverly tucked away: recalling our initial definition of the golden ratio, show that these diminish simply by the golden ratio, compared to the central pentagram. They are therefore smaller than the big pentagram by ³. See if you can show that smallest pentagrams have pentagons at their centres smaller than the outlying one (bounding the pentagram) by ⁵.

Let us now look at the two outer pentagons. The corners of one are on the mid-points of the other, so their sizes will be as cosine of /5 (36°) which is half the golden ratio. So, the very outermost pentagon is larger than the very smallest (there are five of these), by half of the sixth power of the golden ratio. Starting from the smallest ones, the scale of these pentagons was thus increasing as

, ³, ⁵, 2⁴

So, in wheat and barley fields around Avebury in June-July 2003 an astonishing manifestation of powers of the Golden Ratio took place.

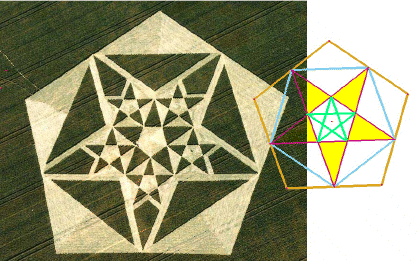This formation developed an expanding series of pentagrams/pentagons, reaching up to the fourth power of phi.

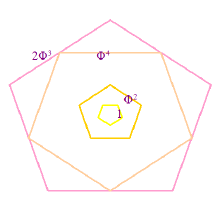
The construction (here in blue), shows how an infinite sequence of pentagrams can be drawn, each one decreasing by a scale-factor of phi.The Circlemakers have shown us the first step in this sequence, so that five pentagrams surround a central one.

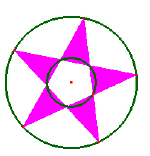
Theorem: if two circles are inscribed inside and outside a regular pentagram, as shown, then their radii are in a phi-squared ratio to each other, i.e one is 2.618. larger than the other. This theorem should enable you to handle all these phi-ratios! The pentagram is a figure which always gives phi-ratios.

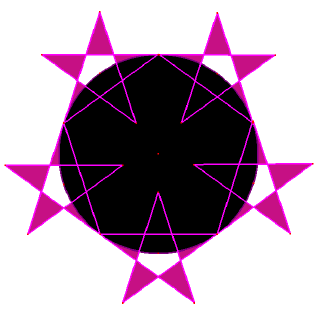
The same Artist, surely, developed this theme, into one of the masterpieces of 2007. This exquisite construction was indeed a work of Art. Its pattern of expanding pentagons and pentagrams shows the same proportion as in the 2003 formation.

Theorem: if five pentagrams are made to touch a central pentagram (i.e. they are smaller in a phi-ratio to it) then their centres lie on the circle surrounding that main pentagram.

Extending the sides of these five pentagrams makes another one at the centre, reduced in a phi-ratio.

This is a quite magical geometry that has been inscribed here. No-one ever imagined this shape before. In each of the five directions there are three prominent parallel lines, and can you show that the distances between them form the phi-ratio? They are, but showing this isn't easy! We may also discern large rhombi, diamond shapes, and these subdivide to make golden parallelograms, whose sides are in the golden ratio.

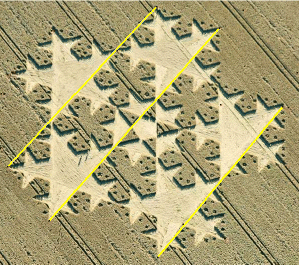
This diagram was drawn by Freddy Silva, based on the geometric insights of Gerald Hawkins. Across the Atlantic, a year or two after the event, he apprehended its basic, double-pentagram architecture - when no-one over here had done. But now, we go a bit further.

If two circles are drawn, one inside a regular pentagon, and the other outside touching its corners; and ten circles are drawn standing on that larger circle, all touching each other: they will have the same size as the circle at the centre.

This is a new symmetry which the Circlemakers are showing to us, a 'golden' theorem. It isn't in books. The large circle turns out to be bigger than these others, by twice the golden ratio, ie 2.

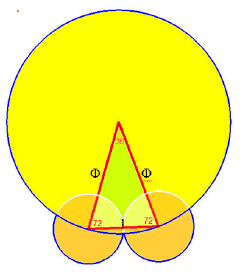The outer Web silhouettes these ten circles, and five ripples emanate from them, making the spider-web. The fifth touches that circle, which passes through the pentagram intersections (This circle is smaller than the outermost circle, by the square of the golden ratio, ²). We note that all these outermost ripples (or innermost webs) cut through the centres of adjacent circles, suggesting that they are double the radius of the primary circle: as the sequence of ripples move through the spider-web, it doubles in size. Thereby they come to just touch that inner Pentagram circle.

Proof: Join up the centre of the formation to the centres of two of the adjacent circles on the perimeter, to make a one-tenth slice of the whole design. This gives us the 'divine triangle' whose top angle is 36° and sides make - ratios to each other. Each of the outer circles have a radius equal to half of the base length, showing that the big and little circles are in a 2 ratio to each other.^[1]

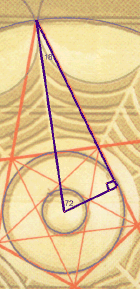Chopping that triangle in half, will make the top angle 18°, with a right-angle at its base and 72° in the other corner. We reconstruct that same triangle, by drawing a line from a pentagram corner to the centre, and also along the line of the pentagram to where it touches that centre circle, as shown. Because this is the same triangle, it will have the same ratio of the side lengths, namely 1:2; and therefore, the radius of the centre circle has to be the same as the outer ones.

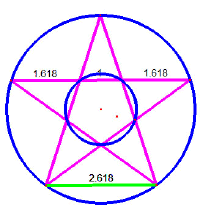The sides of a pentagram 'grow' in golden ratio proportions, as the diagram shows: from unity, as the length of the inner pentagon side, we get to the square of phi along the outer pentagon side (The golden ratio squares by adding one, i.e. ² = + 1). The 2.618 along the bottom is phi-squared. This line is in the same proportion to the outer circle, as is the smaller to the inner circle, and thus the two circles are in a ² ratio of their sizes.

The golden message of this formation, speaks of an interconnection between Inner and Outer. Taking the radius of the big circle as unity, then that of the circle intersecting the pentagram arms will be 1/ ², and the difference between them is 1 - 1/ ² . But, we've seen how the ten surrounding circles have radii 1/2 , and twice that is 1/ . These are equal, i.e. 1 - 1/ ² = 1/ . Can you show that?

This formation contains exquisite inner symmetries. Its web is golden, and pulls us into an organic phi-geometry. The two concentric rings of standing wheat at the centre, are also in a phi-squared ratio. Back in 1994, who knew how to do this golden ratio math? It wasn't in any school math syllabus, it wasn't in textbooks.

Quiz question: can you show, that the innermost circle is smaller than the big, outer circle by twice the cube of the golden ratio?

^[1]   I.e., the cosine of 72° is 1/2

Thanks to Mark Fussell for use of Windmill Hill picture, Zef Damen for Triple pentagram image.